AQA Specification focus:
'- Understanding the rate equation: Rate = k[A]^m[B]^n and its components: rate constant (k), orders of reaction (m, n).
- Skills: Defining and calculating the order of reaction and rate constant, understanding how m and n values influence rate.'
Introduction to the Rate Equation
The rate equation is a mathematical representation that links the speed of a chemical reaction to the concentrations of its reactants through a constant of proportionality known as the rate constant.
The General Form
Rate = k[A]ⁿ[B]ⁿ
In this equation:
Rate refers to the reaction rate, indicating how quickly reactants are converted to products, typically expressed in moles per litre per second (mol L⁻¹ s⁻¹).
k represents the rate constant, a crucial parameter that embodies the reaction's intrinsic speed under given conditions.
[A] and [B] denote the molar concentrations of the reactants A and B, respectively.
m and n are the orders of reaction with respect to reactants A and B, providing insights into how changes in reactant concentrations influence the reaction rate.
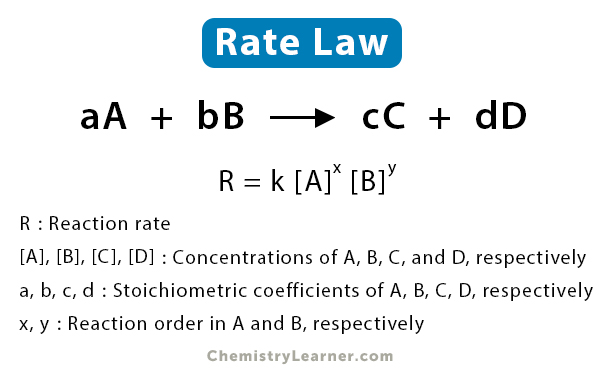
Image courtesy of Chemistry Learner
Delving into the Rate Constant (k)
The rate constant is a pivotal element of the rate equation, shedding light on the reaction's inherent velocity. Its value, determined through experimental means, is influenced by factors such as temperature and the presence of catalysts. The dimensions of k adapt according to the overall reaction order to maintain the rate's units as mol L⁻¹ s⁻¹.
Unpacking Orders of Reaction (m, n)
The reaction order for each reactant unveils the relationship between its concentration and the reaction's rate:
First-order reactions exhibit a proportional relationship between rate and reactant concentration. If the concentration doubles, so does the rate.
Second-order reactions may see the rate proportional to the square of one reactant's concentration or to the product of two reactants' concentrations. Doubling one reactant's concentration quadruples the rate.
Zero-order reactions remain unaffected by the concentration of the reactants, maintaining a constant rate as long as the reactants are present.

Image courtesy of chemistry learner.
Calculating the Order of Reaction
Identifying the order of reaction is a key skill, enabling chemists to understand how reactant concentrations affect the rate.
Initial Rates Method
This approach involves varying the initial concentrations of reactants and observing the initial reaction rate. This method hinges on the premise that initial rates provide a clear window into the reaction's dynamics before significant concentration changes occur.
Graphical Analysis
Graphical methods offer another avenue for deducing reaction orders:
For first-order reactions, a plot of ln[reactant] versus time yields a straight line, indicating a linear relationship.
Second-order reactions manifest as a linear relationship when 1/[reactant] is plotted against time.
Practical Application
Experimental Setup: Create a series of mixtures with different concentrations of one reactant while keeping others constant.
Rate Measurement: Determine the initial rate for each mixture, focusing on the early stages where concentration changes are minimal.
Data Analysis: Plot the data to discern the relationship between rate and reactant concentration, using this to infer the reaction order.
Impact of m and n Values on Rate
The orders of reaction profoundly influence how a chemical reaction progresses, dictating the relationship between reactant concentrations and the reaction rate.
First-Order Reactions: The rate changes linearly with the concentration of one reactant. A twofold increase in concentration results in a twofold increase in rate.
Second-Order Reactions: These reactions show a quadratic relationship with the concentration of one reactant or a linear relationship with the product of two reactants' concentrations. Doubling the concentration of one reactant leads to a fourfold increase in the rate.
Zero-Order Reactions: Rate remains constant regardless of changes in reactant concentration, highlighting a reaction's independence from its reactants' molar quantities.
Determining the Rate Constant
The rate constant (k) can be calculated using the rate equation once the reaction order and reactant concentrations are known.
Example Calculation
Consider a first-order reaction with an observed rate of 0.02 mol L⁻¹ s⁻¹ when the concentration of A is 0.1 mol L⁻¹. The rate constant k is calculated as follows:
Rate = k[A]
Rearranging for k:
k = Rate / [A] = 0.02 mol L⁻¹ s⁻¹ / 0.1 mol L⁻¹ = 0.2 s⁻¹
Key Points to Remember
Ensure consistency in units when calculating k to maintain the integrity of the rate equation.
The rate constant is intrinsic to the reaction and its specific conditions, particularly temperature. Its value offers insights into the reaction's speed and can indicate the presence of catalysts or the effect of temperature changes.
Advanced Considerations
Temperature Dependence of the Rate Constant
The rate constant's sensitivity to temperature variations is a critical aspect of reaction kinetics. The Arrhenius equation, ( k = Ae{-Ea/RT} ), where ( Ea ) is the activation energy and ( A ) the pre-exponential factor, provides a quantitative framework for understanding this relationship.

Image courtesy of Julee Ashmead
Catalysis and Reaction Rates
Catalysts play a transformative role in chemical reactions, offering pathways with lower activation energies. This results in an increased rate constant ( k ), thereby accelerating the reaction without being consumed in the process.
Reaction Mechanisms and Rate Equations
The overall rate equation is a reflection of the reaction mechanism, particularly the slowest step known as the rate-determining step. Understanding the mechanism allows for a more nuanced interpretation of the rate equation, linking molecular events to macroscopic observations.
Practical Applications and Experiments
Laboratory experiments, such as the iodine clock reaction, provide tangible experiences in determining reaction orders and rate constants. These experiments not only reinforce theoretical knowledge but also hone practical skills in data collection and analysis.
In conclusion, mastering the fundamentals of rate equations equips students with the tools to decode the complex interplay of factors governing chemical reactions. This knowledge forms the bedrock upon which further studies in kinetics and broader chemical principles are built, opening avenues for exploration in both academic and industrial contexts.
FAQ
Catalysts play a pivotal role in chemical reactions by providing an alternative pathway with a lower activation energy (Eₐ) for the reaction to proceed. This alternative pathway increases the number of effective collisions between reactant molecules, thereby increasing the rate constant (k) of the reaction. It's important to note that while catalysts increase the rate at which equilibrium is achieved, they do not alter the position of the equilibrium itself.
The overall order of the reaction, however, remains unchanged by the presence of a catalyst. This is because the reaction order is determined by the stoichiometry of the rate-determining step, which is an inherent characteristic of the reaction mechanism itself. The catalyst merely makes the rate-determining step occur more rapidly but does not modify the reaction's fundamental stoichiometric relationships. Therefore, while the presence of a catalyst can significantly increase the rate of a reaction (reflected in a higher rate constant), it does not affect the mathematical expression that relates the reaction rate to the concentrations of the reactants (the rate equation), and thus the overall order of reaction remains the same.
Yes, the order of reaction can indeed be negative, though this is less common than positive or zero orders. A negative order in a rate equation implies that the rate of the reaction decreases as the concentration of the reactant increases. This counterintuitive scenario is often observed in complex reactions where the presence of a reactant inhibits the reaction rate beyond a certain concentration.
For instance, in some reactions involving catalysts, an excess of one of the reactants can lead to the 'poisoning' of the catalyst's active sites, thereby decreasing the rate of the reaction. In such cases, the rate equation might include a term with a negative exponent for the concentration of the excess reactant. This negative order indicates the inhibitory effect that the reactant has on the reaction rate, showcasing the nuanced and sometimes unexpected ways in which reactant concentrations can influence the kinetics of a chemical reaction.
Determining the rate constant (k) experimentally involves measuring the rate of the reaction under controlled conditions and then manipulating the data to fit the form of the rate equation appropriate for the reaction's order. For a reaction of any given order, this process typically involves the following steps:
Initial Rate Method: The initial rate of the reaction is measured for several different initial concentrations of the reactants. This is done by monitoring a property that changes with reaction progress (such as concentration, pressure, or optical absorbance) over a short initial period, ensuring that the reactant concentrations change minimally.
Graphical Analysis: For first-order reactions, a plot of the natural logarithm of reactant concentration versus time should yield a straight line, with the slope equal to -k. For second-order reactions, a plot of the inverse of reactant concentration versus time will be linear, with the slope providing the rate constant. Zero-order reactions are indicated by a linear relationship between reactant concentration and time, with the slope equal to -k.
Curve Fitting: For reactions of more complex orders, or when multiple reactants are involved, the rate data can be fitted to the rate equation using nonlinear regression analysis to extract the rate constant.
This experimental determination of k is crucial for understanding the kinetics of a reaction and can vary significantly with changes in conditions such as temperature, pH, and the presence of a catalyst.
Fractional reaction orders often arise in complex reactions that involve mechanisms more intricate than simple one-step processes. These fractional orders can result from reactions that proceed through multiple steps, where the rate-determining step involves a mechanism that does not straightforwardly relate to the stoichiometry of the overall reaction. For example, in a reaction mechanism involving an intermediate complex that partially breaks down into the final product, the rate of reaction might depend on the concentration of this intermediate in a manner that is not a simple whole number ratio.
Interpreting fractional reaction orders involves understanding that the reaction rate's dependency on a reactant's concentration does not follow a simple direct or square relationship. Instead, it indicates a more nuanced interaction, where the rate increases with the concentration of the reactant to a power that is not an integer. This fractional order provides valuable insight into the reaction mechanism, suggesting the presence of intermediate species or complex rate-determining steps that do not directly correspond to the overall reaction equation.
The concept of the rate-determining step is fundamental in formulating the rate equation for a chemical reaction. The rate-determining step is the slowest step in a reaction mechanism, acting as a bottleneck that controls the overall rate of the reaction. Since the overall reaction cannot proceed faster than this slowest step, the rate equation is formulated based on the kinetics of this particular step.
When constructing the rate equation, the stoichiometry and the reactants involved in the rate-determining step are of primary importance. The orders of reaction in the rate equation correspond to the coefficients of the reactants in this step, not necessarily those in the overall balanced equation for the reaction. This means that even if a reactant appears in the overall reaction, it may not appear in the rate equation if it is not involved in the rate-determining step.
Understanding the rate-determining step allows chemists to predict and manipulate reaction rates more effectively. By altering conditions or concentrations of reactants involved in this crucial step, one can control the reaction speed, which is especially important in industrial processes where efficiency and optimization are key.
Practice Questions
Given the reaction between substance A and substance B is found to have a rate equation of Rate = k[A]²[B], determine the order of reaction with respect to A and B and explain what this implies about the reaction mechanism.
The order of reaction with respect to A is 2, indicating that the rate of reaction is proportional to the square of the concentration of A. This suggests that either two molecules of A are involved in the rate-determining step of the reaction, or the concentration of A significantly affects the reaction rate through a bimolecular interaction. The order of reaction with respect to B is 1, implying that the rate of reaction is directly proportional to the concentration of B, suggesting that one molecule of B is involved in the rate-determining step. These orders imply a complex reaction mechanism where the concentration of A has a more significant effect on the rate than B.
A reaction has a rate constant (k) of 0.030 s⁻¹ at 25°C and an activation energy (Eₐ) of 75 kJ mol⁻¹. Using the Arrhenius equation, explain how the rate constant would change if the temperature were increased to 35°C.
Using the Arrhenius equation, k = Ae(-Eₐ/RT), where A is the Arrhenius constant, Eₐ is the activation energy, R is the gas constant (8.314 J mol⁻¹ K⁻¹), and T is the temperature in Kelvin. Increasing the temperature from 25°C (298 K) to 35°C (308 K) would increase the exponent's value, as the negative sign in the exponent means a higher temperature reduces the magnitude of the negative exponent, leading to a larger value of e(-Eₐ/RT) and thus a higher rate constant. Therefore, the rate constant would increase, indicating that the reaction would proceed faster at the higher temperature.

